IB-MYP 4 & 5
- Description
- Curriculum
The IB-MYP (Middle Years Programme) typically covers a range of math topics that focus on developing problem-solving skills, mathematical reasoning, and understanding of key concepts. The specific syllabus can vary depending on the school, but here’s an overview of common topics covered in Grade 9/10 math under the MYP:
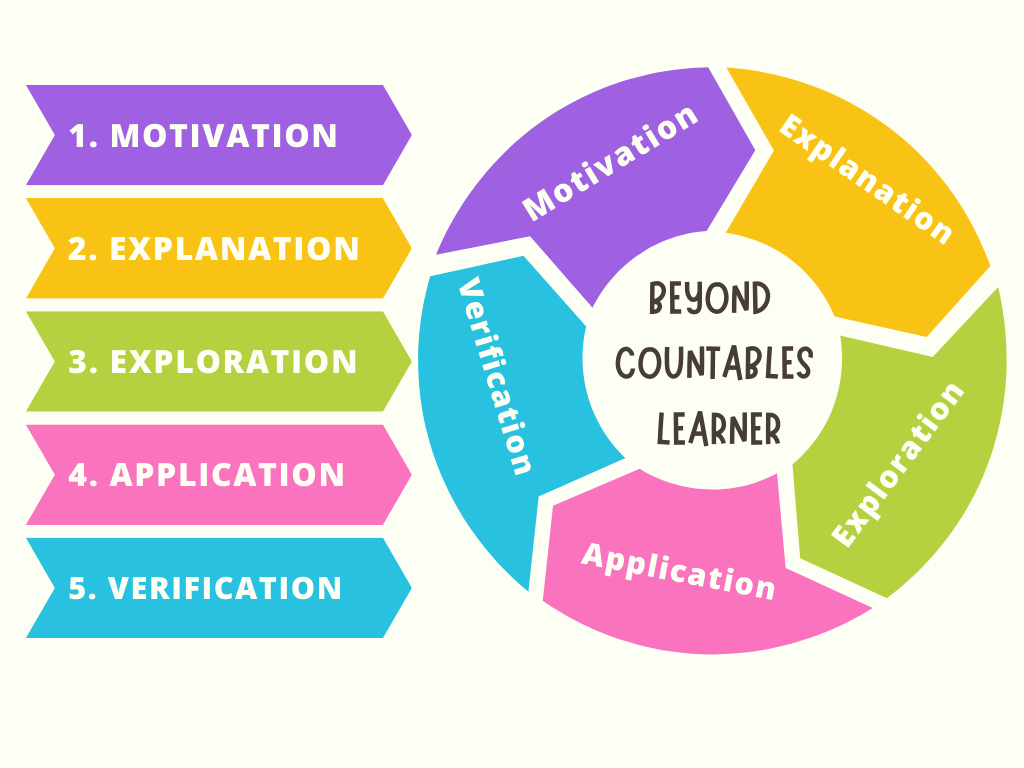
Our Approach to Teaching and Learning:
We follow the 5 step process depicted in the picture below.
The following topics are covered in this course (Ex - EXTENDED ONLY TOPICS and Enr - ENRICHMENT ONLY TOPICS)
-
1
Number- Absolute values
- Inequalities
- Irrational Numbers
- Surds and radicals
- Standard form (Scientific notation)
- Upper and lower bounds (Ex)
- Laws of exponents - positive, negative and rational (Ex)
- Number systems notation
- Direct and inverse proportion
- Number sequences
- Logarithms - laws of logarithms and use of technology (Ex)
- Exponential equations (Enr)
- Rationalizing the denominator (Enr)
- Number bases (Enr)
-
2
Algebra
- Factorizing quadratic expressions
- Solving quadratic equations
- Changing the subject of an equation
- Arithmetic and geometric sequences (Ex)
- Arithmetic and geometric series (Enr)
- Sigma notation (Enr)
- Convergence and divergence of series (Enr)
-
3
Functions and modeling
- Mappings
- Function notation
- Linear functions
- Equation of the form y=mx+c
- Parallel and perpendicular lines
- System of equations
- Quadratic functions
- Algorithms
- Advanced functions (Ex)
- Transformation of quadratic functions (Ex)
- Rational functions (Ex)
- Graphing trigonometric functions (Ex)
- Linear programming (Ex)
- Networks - edges and arcs, vertices/nodes, path (Ex)
- Weighted networks (Ex)
- Domain and Range (Ex)
- Exponential functions (Enr)
- Transforming cubic and trigonometric functions (Enr)
- Logarithmic functions including ln and e (Enr)
- Composite and inverse functions (Enr)
- Probability (Enr)
- Pascal’s triangle (Enr)
-
4
Geometry and Trigonometry
- Metric conversions
- Volume of regular polyhedra
- Volume and capacity of complex shapes (Ex)
- Similarity and congruence
- Distance, midpoint and gradient formulae
- Relationship between gradient of parallel and perpendicular lines (Ex)
- Movement on a plane - isometric transformations, enlargements and tessellations
- Enlargement around a point including rational factor (Ex)
- Rotation around a point
- Circle geometry
- Triangle properties
- Bearings
- Pythagoras theorem
- Trigonometric ratios in right angled triangles
- Converse of Pythagoras theorem (Ex)
- Sine rule and cosine rule including applications (Ex)
- Fractals (Enr)
- Dilation/Stretch (Enr)
- Inscribing and circumscribing shapes (Enr)
- Arc length and sector using radians (Enr)
- Polar coordinates (Enr)
- Vectors and vector spaces (Enr)
-
5
Statistics and Probability
- Sampling techniques
- Graphic representations - bivariate graphs, scatter graphs, box plots, cumulative frequency graphs
- Line of best bit
- Quartiles and percentiles
- Measures of dispersion - interquartile range (IQR)
- Correlation
- Correlation with technology (Ex)
- Relative frequency
- Sets and operations on sets up to three sets
- Probability -Venn diagrams, tree diagrams and sample spaces
- Mutually exclusive events
- Combined events
- Measures of dispersion - standard deviation (SD) (Ex)
- Histograms for continuous data (Ex)
- Addition and multiplication rule of probability (Ex)
- Conditional Probability (Ex)
- Dependent and independent events (Ex)
- Manual calculation of standard deviation and correlation coefficient (Enr)
- Covariance (Enr)
- Interpolation and extrapolation (Enr)
- Confirmation bias (Enr)
- Counting principles - combinatorics and permutations (Enr)
- Factorials (Enr)
- Geometric mean (Enr)
- Regression - interpretation of results (Enr)
